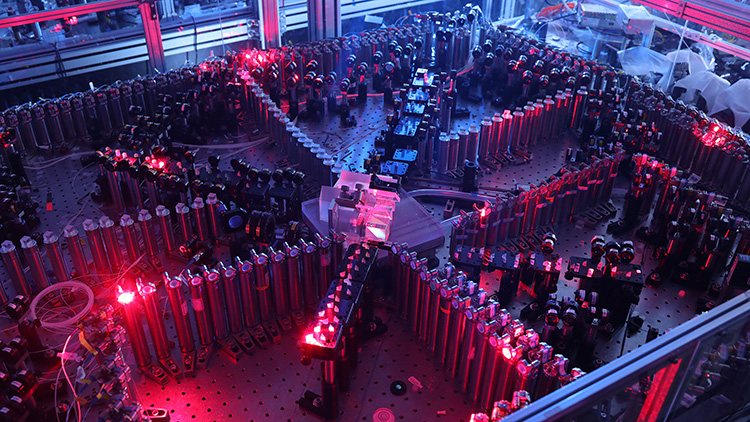
The interferometer part of the experimental setup. The photonic device is realized by sending 50 single-mode squeezed states into a 100-mode interferometer, and then sampling from the output distribution using 100 single-photon detectors.
In 1981, Richard Feynman observed that many-body quantum problems are difficult to solve using classical computers, due to the exponentially growing size of the quantum Hilbert space. He conjectured that quantum computers could solve certain tasks exponentially faster than the classical computers.1 Such a conjecture, of course, would require stringent experimental proof. During the past year, we demonstrated such a quantum advantage, using an all-photonic quantum-computing setup.2
Our demonstration involved a test that has been proposed, based on plausible computational-complexity arguments, for a strict demonstration of quantum computational advantage: boson sampling.3 Interestingly, while boson sampling is much easier to implement experimentally than, for example, Shor’s algorithm on a fault-tolerant quantum computer, it is potentially more compelling as an indicator of computational complexity, as the underlying problem is believed to be of the computationally difficult #P-hard class.
Early demonstrations of boson sampling using quantum-dot single-photon sources culminated at 14-photon detection,4 which can still be simulated on classical computers; thus, these demonstrations did not demonstrate a quantum advantage. In our recent work, we instead performed Gaussian boson sampling5 by sending 50 single-mode squeezed states, with high indistinguishability and squeezing parameters, into a 100-mode ultra-low-loss interferometer with full connectivity and random transformation, and sampled the output using 100 high-efficiency single-photon detectors.2 The whole optical setup was phase-locked to maintain a high coherence between the superposition of all photon number states.
This photonic quantum computer generated up to 76 output photon clicks, which yielded an output state-space dimension of 1030 and a sampling rate that was approximately 1014 faster than using the state-of-the-art simulation strategy and supercomputers. The obtained samples were validated against plausible hypotheses exploiting thermal states, distinguishable photons and uniform distribution to confirm the computational results.
In addition to demonstrating quantum advantage, our Gaussian boson-sampling demonstration links to several potentially practical applications such as graph optimization, quantum chemistry and quantum machine learning. A next step will be to use the machine as a special-purpose photonic platform to investigate these algorithms, as a step toward noisy intermediate-scale quantum processing.
Researchers
H.-S. Zhong, H. Wang, Y.-H. Deng, M.-C. Chen, J. Qin, L.-C. Peng, Y.-H. Luo, D. Wu, X. Jiang, L. Li, N.-L. Liu, C.-Y. Lu and J.-W. Pan, University of Science and Technology of China, China
References
1. R.P. Feynman. Int. J. Theor. Phys. 21, 467 (1982).
2. H.S. Zhong et al. Science 370, 1460 (2020).
3. S. Aaronson and A. Arkhipov, in Proc. 43rd Ann. Symp. on Theory of Computing (2011).
4. H. Wang et al. Phys. Rev. Lett. 123, 250503 (2019).
5. C.S. Hamilton et al. Phys. Rev. Lett. 119, 170501 (2017).